Proposition 31 Problem 23

Find the place of a body moving in an elliptic trajectory

Let:

• A the principal vertex
• S the focus
• O the centre of the ellipsis APB
• P is the place where the body is found

Produce OA to G so as OG may be to OA as OA to OS. Erect the perpendicular GH.

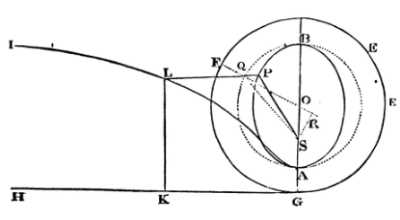
Around bout the centre O, with the interval OG, describe the circle GEF; and on the ruler GH, as a base, suppose the wheel GEF to move forwards, revolving about its axis, and in the mean time by its point A describing the cycloid ALI. Which done, take GK to the perimeter GEFG of the wheel, in the ratio of the time in which the body proceeding from A described the arc AP, to the time of a whole revolution in the ellipsis. Erect the perpendicular KL meeting the cycloid in L; then LP drawn parallel to KG will meet the ellipsis in P, the required place of the body.

For about the centre O with the interval OA describe the semi-circle AQB, and let LP, produced, if need be, meet the arc AQ in Q, and join SQ, OQ. Let OQ meet the arc EFG in F, and upon OQ let fall the perpendicular SR. The area APS is as the area AQS, that is, as the difference between the sector OQA and the triangle OQS, or as the difference of the rectangles ½OQ

…

that is, because ½OQ is given, as the difference between the arc AQ and the right line SR; and therefore (because of the equality of the given ratios SR to the sine of the arc AQ, OS to OA, OA to OG, AQ to GF; and by division, AQ - SR to GF - sine of the arc AQ) as GK, the difference between the arc GF and the sine of the arc AQ. Q.E.D.